Consider a specimen of undistorted austenite occupying some
domain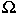 
in 3-dimensional
real space.
The transformation to martensite is through a homogeneous
deformation y = Fx with x in
-
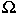
This deformation is mapping from a Bravais lattice of the parent phase to a Bravais lattice of the product phase. The deformation gradient F is found from experimental observations of the lattice parameters of the two phases and the change in symmetry.
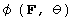with deformation gradient F in
,
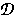where 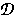is some subset of the set of
second order tensors, L(3,3), with positive determinant and
temperature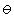 
in
I, a subset of the positive real line.
The free energy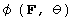
must satisfy certain invariance requirements:
-
frame indifference:
= 
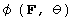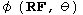for all R in SO(3) -
the set of proper rotations, and -
material symmetry:
= 
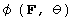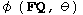for all Q in
Pa
- the point group of the austenite lattice.
From the Polar Decomposition Theorem, the deformation gradient F can be written as RU, where R is a rotation and U is a positive-definite symmetric matrix.
From frame indifference, the free energy depends only upon the symmetric part U of the deformation gradient F. This matrix U is called the Bain strain, lattice distortion or transformation stretch matrix; while,
from material symmetry, there are other deformations which take the austenite lattice into a martensite lattice. These are the variants of the martensite phase.