Other microstructures are possible using both of the phases, and these involve fitting multiple austenite-martensite microstructures together. One such microstructure is the wedge microstructure, where the wedge is of interest because it can provide a mechanism by which a specimen can more easily transform. In fact, a wedge can grow from a line on either a free surface or a grain boundary. Moreover, the wedge is a special microstructure in that it is only possible in materials with special lattice parameters; that is, not all materials which can form an austenite-martensite microstructure can form the wedge; rather, only those materials with lattice parameters which satisfy some special relationship can exhibit the wedge.
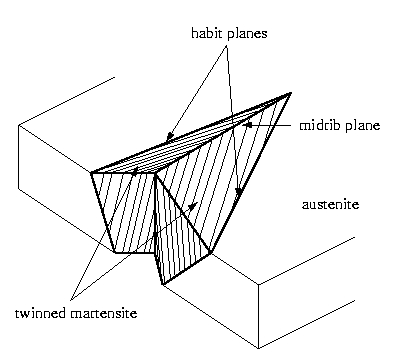
A schematic of a wedge microstructure made from two austenite twinned martensite microstructures is shown in Figure 1 below, where the plane separating the two twinned martensite regions is called a midrib plane.

Figure 1: Schematic of a wedge microstructure made from two austenite twinned martensite microstructures.
I have considered two kinds of wedge microstructures:
Single variant wedge: The single variant wedge is formed by two austenite single variant of martensite microstructures with habit planes that intersect and the corresponding martensite regions also forming a compatible deformation. The single variant wedge produces traces of the habit and midrib planes on the surface of the specimen as depicted in Figure 2 below:
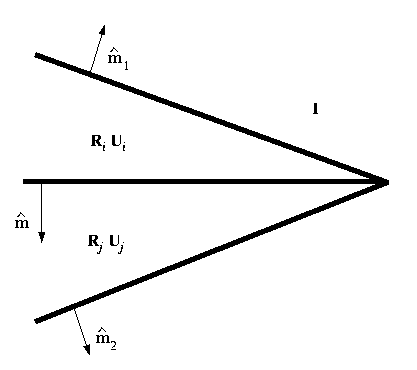
Figure 2: Single variant wedge microstructure, where the straight lines are the intersection of the habit planes and midrib plane with the plane of the page.
There are three compatibility equations which need to be satisfied in order that single variant wedge forms a continuous deformation. Two of the compatibility equations are for the habit planes, while the remaining compatibility equation is along the midrib plane - notice that this equation is just the twinning equation. Necessary and sufficient conditions that all of these equations are satisfied is that the two shape strains from the austenite martensite microstructures are parallel and the corresponding habit plane normals are not parallel.The symmetry amongst the habit plane solutions for the austenite single variant of martensite microstructure can be used to find restrictions on the components of the shape strain and habit plane normal vectors in order to able to form the wedge. These restrictions are given in the following tables.
These conditions give that the single variant wedge is possible if and only if a material has lattice parameters satisfying a special relation. Various special relations have been found for several different transformations as listed in the table below.
Twinned wedge: The twinned wedge is formed by two austenite twinned martensite microstructures with the two habit planes intersecting and the corresponding twinned martensite regions also forming a compatible deformation. The twinned wedge produces traces of the twin, habit, and midrib planes on the surface of the specimen as depicted in Figure 3 below:
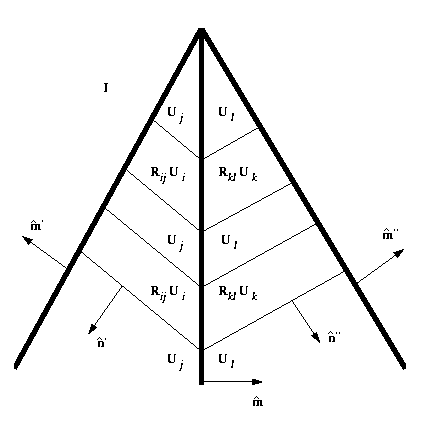
Figure 3: Twinned wedge microstructure, where the straight lines are the intersection of the twin and habit planes and midrib plane with the plane of the page.
There are five compatibility equations which need to be satisfied in order that twinned wedge forms a continuous deformation. These are; two equations along the twin plane; two equations along the habit planes; and one along the midrib plane. As for the single variant wedge, all of these compatibility equations are satisfied if and only if the two shape strains from the austenite martensite microstructures are parallel and the corresponding habit plane normals are not parallel (see [1]).
As above, the symmetry amongst the habit plane solutions for the austenite twinned martensite microstructures can be used to find restrictions on the components of the shape strain and habit plane normal vectors in order to able to form the wedge. These restrictions are given in the following tables. Further, these conditions hold if and only if the lattice parameters satisfy a special relation. Various special relations have been found for several different transformations as listed in the table below.
Various single variant wedge microstructures are possible for different transitions as listed in the table below.
Single Variant Wedge Microstructures |
||
|---|---|---|
| Transition | Number | Observed |
| Cubic-to-Trigonal | 0 | not possible |
| Cubic-to-Tetragonal | 0 | not possible |
| Cubic-to-Orthorhombic | 18 with 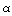 =1
and =1
and 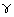 =
f( =
f(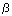 )
(*) )
(*) |
not observed |
| " | 12 with 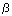 =1
and =1
and 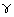 =
g( =
g(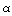 )
(*) )
(*) |
not observed |
| Cubic-to-Monoclinic | 0 | not possible in a particular Ti-Ni alloy (**) |
In the table above, the first column is the transition; the second column lists the number of unique microstructures which can be formed; none of these microstructures have been observed in experiments.
(*) Note: for the cubic to orthorhombic transition, the single variant wedge is possible if and only if one of the transformation stretches describing the transition is equal to one, and the remaining two satisfy some algebraic condition denoted by the functions f and g. Explicit functions are given in [2].
(**) Note: for the cubic to monoclinic transition, the single variant wedge is not possible for a particular Ti-Ni alloy, but restrictions as found for the orthorhombic transition can be found as well. It is expected that the collection of single variant wedges in this transition is very rich.
Various twinned wedge microstructures are possible for different transitions as listed in the table below.
Twinned Wedge Microstructures |
|||
|---|---|---|---|
| Transition | Twin type | Number | Observed |
| Cubic-to-Trigonal | Compound | 12 with 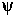 =
116.4 degrees =
116.4 degrees |
unphysical |
| Cubic-to-Tetragonal | Compound | 12 with 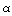 =
h( =
h(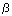 )
(***) )
(***) |
Ni-Al, Ni-Mn, Fe-Ni-C |
| Cubic-to-Orthorhombic | Compound | 12 with 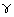 =
i( =
i(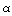 , ,
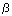 )
(****) )
(****) |
not observed |
| " | Compound | 12 with 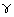 =
j( =
j(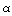 , ,
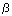 )
(****) )
(****) |
not observed |
| " | Type I | 12 on surfaces (****) | Cu-Al-Ni |
| " | Type II | 12 on surfaces (****) | Cu-Al-Ni |
| " | Type I & Type II (mixed twin) | 24 on curve (****) | not observed |
| Cubic-to-Monoclinic | Type I | 12 | possible in Ti-Ni, not observed (*****) |
| " | Type II | 12 | " |
In the table above, the first column is the transition; the second lists the twin type for which solutions to the habit plane equation are possible; the third column lists the number of unique microstructures which can be formed; the last column gives some alloys for which the various possible wedges have been observed.
(***) Note: for the cubic to tetragonal transition, the explicit function which the transformation stretches must satisfy is given in [1].
(****) Note: for the cubic to orthorhombic transition, the explicit functions or numerically computed surfaces and curves can be found in [2].
(*****) Note: for the cubic to monoclinic transition, there should be a number of surfaces defined by three parameters on which the twinned wedge is possible.
Some references are
Top of this page.
Back to main page.