••1992••
(1)
D. D. Joseph. 1992.
Bernoulli equation and the competition of elastic and inertial pressures in the
potential flow of a second-order fluid,
J.Non-Newtonian Fluid Mech., 42, 385-389.
Abstract
A Bernoullis equation for potential flow of a second order fluid is derived. This equation is used to form an expression for normal extensional stresses at points of stagnation, in which elastic and inertial pressures complete.
Keywords: Bernoullis equation; normal extensional stresses; second order fluid
••1993••
(2)
D. D. Joseph, T. Y. Liao and H. H. Hu. 1993.
Drag and Moment in Viscous Potential Flow,
Eur. J. Mech.
B/Fluids, 12(1), 97-106.
Abstract
We consider solutions of the Navier-Stokes equations in
which the velocity is given by the gradient of a potential. We show that the
drag on bodies and bubbles is the same in viscous and inviscid potential flow.
The lift on two-dimensional bodies is given by the usual Kutta condition but
the moment about the origin of the stresses acting on the body is given by 
where 
is the viscosity, 
is the circulation and 
is the usual moment for an inviscid fluid.
••1994••
(3)
D. D. Joseph and T. Y. Liao. 1994.
Viscous and Viscoelastic Potential Flow,
Trends and
Perspectives in Applied Mathematics, Applied Mathematical Sciences, Sirovich, Arnol'd, eds, Springer-Verlag. Also in
Army HPCRC preprint 93-010., 100, 1-54.
Abstract
Potential
flows of incompressible fluids admit a pressure (Bernoulli) equation when the
divergence of the stress is a gradient as in inviscid
fluids, viscous fluids, linear viscoelastic fluids and second-order fluids. We
show that the equation balancing drag and acceleration is the same for all
these fluids independent of the viscosity or any viscoelastic parameter and
that the drag is zero in steady flow. The unsteady drag on bubbles in a viscous
(and possibly in a viscoelastic) fluid may be approximated by evaluating the
dissipation integral of the approximating potential flow because the neglected
dissipation in the vorticity layer at the
traction-free boundary of the bubble gets smaller as the Reynolds number is
increased. Using the potential flow approximation, the drag 
on
a spherical gas bubble of radius 
rising
with velocity 
in a linear
viscoelastic liquid of density 
and shear modules 
is given by
and in a
second-order fluid by
where 
is the coefficient of
the first normal stress and 
is the viscosity of the fluid. Because 
is negative, we see from this formula that
the unsteady normal stresses oppose inertia; that is, oppose the acceleration
reaction. When is slowly varying, the
two formulas coincide. For steady flow, we obtain 
for both viscous and
viscoelastic fluids. In the case where the dynamic contribution of the interior
flow of the bubble cannot be ignored as in the case of liquid bubbles, the
dissipation method gives an estimation of the rate of total kinetic energy of
the flows instead of the drag. When the dynamic effect of the interior flow is
negligible but the density is important, this formula for the rate of total
kinetic energy leads to 
where 
is
the density of the fluid (or air) inside the bubble and 
is the volume of the
bubble.
Classical
theorems of vorticity for potential flow of ideal
fluids hold equally for viscous and viscoelastic fluids. The drag and lift on
two-dimensional bodies of arbitrary cross section in viscoelastic potential
flow are the same as in potential flow of an inviscid
fluid but the moment 
in
a linear viscoelastic fluid is given by
where 
is
the inviscid moment and 
is the circulation,
and
in a second-order
fluid. When is slowly varying, the
two formulas for coincide.
For steady flow, they reduce to
which is also the
expression for in
both steady and unsteady potential flow of a viscous fluid.
Potential
flows of models of a viscoelastic fluid like Maxwell's are studied. These
models do not admit potential flows unless the curl of the divergence of the
extra-stress vanishes. This leads to an over-determined system of equations for
the components of the stress. Special potential flow solutions like uniform
flow and simple extension satisfy these extra conditions automatically but
other special solutions like the potential vortex can satisfy the equations for
some models and not for others.
(4)
D. D. Joseph and T. Y. Liao. 1994.
Potential Flow of Viscous and Viscoelastic Fluids,
J. Fluid Mech.,
265,
1-23.
Abstract
Potential flows of
incompressible fluids admit a pressure (Bernoulli) equation when the divergence
of the stress is a gradient as in inviscid fluids,
viscous fluids, linear viscoelastic fluids and
second-order fluids. We show that in potential flow without boundary layers the
equation balancing drag and acceleration is the same for all these fluids,
independent of the viscosity or any viscoelastic
parameter, and that the drag is zero when the flow is steady. But, if the
potential flow is viewed as an approximation to the actual flow field, the
unsteady drag on bubbles in a viscous (and possibly in a viscoelastic)
fluid may be approximated by evaluating the dissipation integral of the
approximating potential flow because the neglected dissipation in the vorticity layer at the traction-free boundary of the bubble
gets smaller as the Reynolds number is increased. Using the potential flow approximation,
the actual drag 
on a spherical gas
bubble of radius 
rising with velocity 
in a linear viscoelastic liquid of density 
and shear modules 
is estimated to be
,
and, in a second-order fluid,
,
where 
is the coefficient of
the first normal stress and 
is the viscosity of
the fluid. Because 
is negative, we see
from this formula that the unsteady normal stresses oppose inertia; that is,
oppose the acceleration reaction. When is slowly varying, the
two formulae coincide. For steady flow, we obtain the approximate drag 
for both viscous and viscoelastic fluids. In the case where the dynamic contribution
of the interior flow of the bubble cannot be ignored as in the case of liquid
bubbles, the dissipation method gives an estimation of the rate of total
kinetic energy of the flows instead of the drag. When the dynamic effect of the
interior flow is negligible but the density is important, this formula for the
rate of total kinetic energy leads to 
where 
is the density of the
fluid (or air) inside the bubble and 
is the volume of the
bubble.
Classical theorems of vorticity for potential flow of ideal fluids hold equally
for second-order fluid. The drag and lift on two-dimensional bodies of
arbitrary cross-section in a potential flow of second-order and linear viscoelastic fluids are the same as in potential flow of an
inviscid fluid but the moment 
in a linear viscoelastic fluid is given by
,
where 
is the inviscid moment and 
is the circulation,
and
,
in a second-order fluid. When
is slowly varying, the
two formulae for coincide. For steady
flow, they reduce to
,
which is also the expression
for in both steady and
unsteady potential flow of a viscous fluid. Moreover, when there is no stream,
this moment reduces to the actual moment 
on a rotating rod.
Potential flows of models of
a viscoelastic fluid like Maxwell's are studied.
These models do not admit potential flows unless the curl of the divergence of
the extra stress vanishes. This leads to an over-determined system of equations
for the components of the stress. Special potential flow solutions like uniform
flow and simple extension satisfy these extra conditions automatically but
other special solutions like the potential vortex can satisfy the equations for
some models and not for others.
••1999••
(5)
D. D. Joseph, J. Belanger and G. S. Beavers. 1999.
Breakup of a liquid drop suddenly exposed to a high-speed airstream,
Int. J. Multiphase Flow, 25, 1263-1303.
Abstract
The breakup of viscous and viscoelastic drops in the
high speed airstream behind a shock wave in a shock tube was photographed with
a rotating drum camera giving one photograph every 
. From these photographs we created movies of the fragmentation
history of viscous drops of widely varying viscosity, and viscoelastic drops,
at very high Weber and Reynolds numbers. Drops of the order of one millimeter
are reduced to droplet clouds and possibly to vapor in times less than 
. The movies may be viewed at
http://www.aem.umn.edu/research/Aerodynamic_Breakup. They reveal sequences of
breakup events which were previously unavailable for study. Bag and
bag-and-stamen breakup can be seen at very high Weber numbers, in the regime of
breakup previously called 'catastrophic'. The movies allow us to generate
precise displacement-time graphs from which accurate values of acceleration (of
orders 104 to 105 times the acceleration of gravity) are
computed. These large accelerations from gas to liquid put the flattened drops
at high risk to Rayleigh-Taylor instabilities. The most unstable
Rayleigh-Taylor wave fits nearly perfectly with waves measured on enhanced
images of drops from the movies, but the effects of viscosity cannot be neglected.
Other features of drop breakup under extreme conditions, not treated here, are
available on our Web site.
••2001••
(6)
T. Funada and D. D. Joseph. 2001.
Viscous potential flow analysis of Kelvin-Helmholtz instability in a channel,
J. Fluid
Mech., 445, 263-283.
Abstract
We study the stability of stratified gas-liquid flow in
a horizontal rectangular channel using viscous potential flow. The analysis
leads to an explicit dispersion relation in which the effects of surface
tension and viscosity on the normal stress are not neglected but the effect of
shear stresses are neglected. Formulas for the growth rates, wave speeds and
neutral stability curve are given in general and applied to experiments in
air-water flows. The effects of surface tension are always important and actually
determine the stability limits for the cases in which the volume fraction of
gas is not too small. The stability criterion for viscous potential flow is
expressed by a critical value of the relative velocity. The maximum critical
value is when the viscosity ratio is equal to the density ratio; surprisingly
the neutral curve for this viscous fluid is the same as the neutral curve for
inviscid fluids. The maximum critical value of the velocity of all viscous
fluids is given by inviscid fluids. For air at 20oC and liquids with density 
g/cm3 the liquid viscosity for the critical
conditions is 15 cp; the critical velocity for liquids with viscosities larger
than 15 cp are only slightly smaller but the critical velocity for liquids with
viscosities smaller than 15 cp, like water, can be much lower. The viscosity of
the liquid has a strong affect on the growth rate. The viscous potential flow
theory fits the experimental data for air and water well when the gas fraction
is greater than about 70%.
(7)
T. W. Pan, D. D. Joseph and R. Glowinski. 2001.
Modelling Rayleigh-Taylor instability of a sedimenting suspension of several thousand circular particles in a direct numerical simulation,
J. Fluid Mech., 434, 23-37.
Abstract
In this paper we study the sedimentation of several thousand circular particles in two dimensions using the method of distributed Lagrange multipliers for solid-liquid flow. The simulation gives rise to fingering which resembles Rayleigh-Taylor instabilities. The waves have a well-defined wavelength and growth rate which can be modeled as a conventional Rayleigh-Taylor instability of heavy fluid above light. The heavy fluid is modelled as a composite solid-liquid fluid with an effective composite density and viscosity. Surface tension cannot enter this problem and the characteristic shortwave instability is regularized by the viscosity of the solid-liquid dispersion. The dynamics of the Rayleigh{Taylor instability are studied using viscous potential flow, generalizing work of Joseph, Belanger & Beavers (1999) to a rectangular domain bounded by solid walls; an exact solution is obtained.
••2002••
(8)
D. D. Joseph, G. S. Beavers and T. Funada. 2002.
Rayleigh-Taylor instability of viscoelastic drops at high Weber numbers,
J. Fluid Mech., 453, 109-132.
Abstract
Movies of the breakup of viscous and viscoelastic
drops in the high speed airstream behind a shock wave in a shock tube have been
reported by Joseph, Belanger and Beavers (1999). They performed a Rayleigh-Taylor stability
analysis for the initial breakup of a drop of Newtonian liquid and found that the most unstable Rayleigh-Taylor wave fits nearly perfectly with waves measured on enhanced images of drops from the movies, but the effects of viscosity cannot be neglected. Here we construct a Rayleigh-Taylor stability analysis for an Oldroyd B fluid using measured data
for acceleration, density, viscosity and relaxation time 
. The most unstable wave is a sensitive function
of the retardation time 
which fits
experiments when 
. The growth rates for the most unstable wave are
much larger than for the comparable viscous drop, which agrees with the
surprising fact that the breakup times for viscoelastic drops are shorter. We
construct an approximate analysis of Rayleigh-Taylor instability based on
viscoelastic potential flow which gives rise to nearly the same dispersion
relation as the unapproximated analysis.
(9)
T. Funada and D. D. Joseph. 2002.
Viscous potential flow analysis of capillary instability,
Int. J. Multiphase Flow,
28(9), 1459-1478.
Abstract
Capillary instability of a
viscous fluid cylinder of diameter 
surrounded by another
fluid is determined by a Reynolds number 
, a viscosity ratio 
and a density ratio 
. Here 
is the capillary
collapse velocity based on the more viscous liquid which may be inside or
outside the fluid cylinder. Results of linearized analysis based on potential
flow of a viscous and inviscid fluid are compared with the unapproximated
normal mode analysis of the linearized Navier-Stokes equations. The growth
rates for the inviscid fluid are largest, the growth rates of the fully viscous
problem are smallest and those of viscous potential flow are between. We find that
the results from all three theories converge when 
is large with
reasonable agreement between viscous potential and fully viscous flow with 
. The convergence results apply to two liquids as well as to
liquid and gas.
••2003••
(10)
D. D. Joseph. 2003.
Viscous potential flow,
J. Fluid
Mech., 479, 191-197.
Abstract
Potential
flows 
are solutions of the Navier-Stokes equations for viscous incompressible fluids
for which the vorticity is identically zero. The
viscous term 
vanishes, but the
viscous contribution to the stress in an incompressible fluid (Stokes 1850)
does not vanish in general. Here, we show how the viscosity of a viscous fluid
in potential flow away from the boundary layers enters Prandtl's
boundary layer equations. Potential flow equations for viscous compressible fluids
are derived for sound waves which perturb the Navier-Stokes
equations linearized on a state of rest. These linearized equations support a potential flow with the
novel features that the Bernoulli equation and the potential as well as the
stress depend on the viscosity. The effect of viscosity is to produce decay in
time of spatially periodic waves or decay and growth in space of time-periodic
waves.
In
all cases in which potential flows satisfy the Navier-Stokes
equations, which includes all potential flows of incompressible fluids as well
as potential flows in the acoustic approximation derived here, it is neither
necessary nor useful to put the viscosity to zero.
(11)
D. D. Joseph. 2003.
Rise velocity of spherical cap bubble,
J. Fluid
Mech., 488, 213-233.
Abstract
The
theory of viscous potential flow is applied to the problem of finding the rise
velocity
of a spherical cap bubble
(see Davies & Taylor 1950; Batchelor 1967). The rise velocity is given by
,
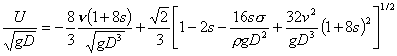where
is the radius of the cap, 
and 
are the density and kinematic viscosity of the liquid, 
is surface tension, 
and 
is the deviation of the
free surface from perfect sphericity 
near the stagnation point 
. The bubble nose is more pointed when 
and blunted when 
. A more pointed bubble increases the rise velocity; the blunter bubble
rises slower. The Davies & Taylor (1950) result arises when 
and vanish; if alone is zero,
,
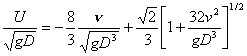showing
that viscosity slows the rise velocity. This equation gives rise to a
hyperbolic drag law
,
which agrees with data on the rise velocity of spherical cap bubbles given by Bhaga & Weber (1981).
(12)
J. Wang and D. D. Joseph. 2003.
Potential flow of a second order fluid over a sphere or an ellipse,
J. Fluid
Mech., 511, 201-215.
Abstract
We study the potential flow of a second-order fluid over a sphere or an ellipse. The normal stress at the surface of the body is calculated and has contributions from the inertia, viscous and viscoelastic effects. We investigate the effects of Reynolds number and body size on the normal stress; for the ellipse, various angles of attack and aspect ratios are also studied. The effect of the viscoelastic terms is opposite to that of inertia; the normal stress at a point of stagnation can change from compression to tension. This causes long bodies to turn into the stream and causes spherical bodies to chain. For a rising gas bubble, the effect of the viscoelastic and viscous terms in the normal stress is to extend the rear end so that it tends to the cusped trailing edge observed in experiments.
(13)
T. Funada and D. D. Joseph. 2003.
Viscoelastic potential flow analysis of capillary instability,
J. Non-Newtonian Fluid Mech., 111, 87-105.
Abstract
Analysis of the linear theory of capillary instability of threads of
Maxwell fluids of diameter D is carried out for the
unapproximated normal mode solution and for a solution based on viscoelastic
potential flow. The analysis here extends the analysis of viscous potential
flow [Int. J. Multiphase Flow 28 (2002) 1459] to viscoelastic fluids
of Maxwell type. The analysis is framed in dimensionless variables with a
velocity scale based on the natural collapse velocity 
(surface tension/liquid viscosity). The collapse
is controlled by two dimensionless parameters, a Reynolds number 
where Oh is
the Ohnesorge number, and a Deborah number 
where 
is the relaxation time. The density ratio 
and 
are nearly zero and do not have a significant
effect on growth rates. The dispersion relation for viscoelastic potential flow
is cubic in the growth rate 
and it can be solved explicitly and computed
without restrictions on the Deborah number. On the other hand, the iterative
procedure used to solve the dispersion relation for fully viscoelastic flow
fails to converge at very high Deborah number. The growth rates in both
theories increase with Deborah number at each fixed Reynolds number, and all
theories collapse to inviscid potential flow (IPF) for any fixed Deborah number
as the Reynolds number tends to infinity.
Keywords: Instability;
Capillary; Viscoelastic; Viscous; Inviscid; Oldroyd
••2004••
(14)
D. D. Joseph and J. Wang. 2004.
The dissipation approximation and viscous potential flow,
J. Fluid
Mech., 505, 365-377.
Abstract
Dissipation approximations have been used to calculate the drag on bubbles and drops and the decay rate of free gravity waves on water. In these approximations, viscous effects are calculated by evaluating the viscous stresses on irrotational flows. The pressure is not involved in the dissipation integral, but it enters into the power of traction integral, which equals the dissipation. A viscous correction of the irrotational pressure is needed to resolve the discrepancy between the zero-shear-stress boundary condition at a free surface and the non-zero irrotational shear stress. Here we show that the power of the pressure correction is equal to the power of the irrotational shear stress. The viscous pressure correction on the interface can be expressed by a harmonic series. The principal mode of this series is matched to the velocity potential and its coefficient is explicitly determined. The other modes do not enter into the expression for the drag on bubbles and drops. They vanish in the case of free gravity waves.
(15)
T. Funada, D. D. Joseph and S. Yamashita. 2004.
Stability of a liquid jet into incompressible gases and liquids,
Int. J.
Multiphase Flow, 30, 1279-1310.
Abstract
We carry out an analysis of the stability of a liquid jet into a gas or another liquid using viscous potential flow. The instability may be driven by Kelvin-Helmholtz KH instability due to a velocity difference and a neckdown due to capillary instability. Viscous potential flow is the potential flow solution of Navier-Stokes equations; the viscosity enters at the interface.
KH instability is induced by a discontinuity of velocity at a gas-liquid interface. Such discontinuities cannot occur in the flow of viscous fluids. However, the effects of viscous extensional stresses can be obtained from a mathematically consistent analysis of the irrotational motion of a viscous fluid carried out here. An explicit dispersion relation is derived and analyzed for temporal and convective/absolute (C/A) instability. We find that for all values of the relevant parameters, there are wavenumbers for which the liquid jet is temporally unstable. The cut-off wavenumber and wavenumber of maximum growth are most important; the variation of these quantities with the density and viscosity ratios, the Weber number and Reynolds is computed and displayed as graphs and asymptotic formulas. The instabilities of a liquid jet are due to capillary and KH instabilities. We show that KH instability cannot occur in a vacuum but capillary instability can occur in vacuum.We present comprehensive results, based on viscous potential flow, of the effects of the ambient.
Temporally unstable liquid jet flows can be analyzed for spatial instabilities by C/A theory; they are either convectively unstable or absolutely unstable depending on the sign of the temporal growth rate at a singularity of the dispersion relation. The study of such singularities is greatly simplified by the analysis here which leads to an explicit dispersion relation; an algebraic function of a complex frequency and complex wavenumber. Analysis of this function gives rise to an accurate Weber-Reynolds criterion for the border between absolute and convective instabilities. Some problems of the applicability to physics of C/A analysis of stability of spatially uniform and nearly uniform flows are discussed.
Keywords: Viscous potential flow; Kelvin-Helmholtz instability; Capillary instability; Temporal instability; Absolute and conveetive instability
(16)
T. Funada, D. D. Joseph, T. Maehara and S. Yamashita. 2004.
Ellipsoidal model of the rise of a Taylor bubble in a round tube,
Int. J. Multiphase Flow, 31, 473-491.
Abstract
The rise velocity of long gas bubbles (Taylor bubbles) in round tubes is modeled by an ovary ellipsoidal cap bubble rising in an irrotational flow of a viscous liquid. The analysis leads to an expression for the rise velocity which depends on the aspect ratio of the model ellipsoid and the Reynolds and Eotvos numbers. The aspect ratio of the best ellipsoid is selected to give the same rise velocity as the Taylor bubble at given values of the Eotvos and Reynolds numbers. The analysis leads to a prediction of the shape of the ovary ellipsoid which rises with same velocity as the Taylor bubble.
••2005••
(17)
J. Wang, D. D. Joseph and T. Funada. 2005.
Purely irrotational theories of the effects of viscosity and viscoelasticity on capillary instability of a liquid cylinder,
J.Non-Newtonian Fluid Mech., 129, 106-116.
Abstract
Capillary instability of a liquid cylinder can arise when either the interior or exterior fluid is a gas of negligible density and viscosity. The shear stress must vanish at the gas-liquid interface but it does not vanish in irrotational flows. Joseph and Wang (2004) derived an additional viscous correction to the irrotational pressure. They argued that this pressure arises in a boundary layer induced by the unphysical discontinuity of the shear stress. Wang, Joseph and Funada (2005) showed that the dispersion relation for capillary instability in the Newtonian case is almost indistinguishable from the exact solution when the additional pressure contribution is included in the irrotational theory. Here we extend the formulation for the additional pressure to potential flows of viscoelastic fluids in flows governed by linearized equations, and apply this additional pressure to capillary instability of viscoelastic liquid filaments of Jeffreys type. The shear stress at the gas-liquid interface cannot be made to vanish in an irrotational theory, but the explicit effect of this uncompensated shear stress can be removed from the global equation for the evolution of the energy of disturbances. This line of thought allows us to present the additional pressure theory without appeal to boundary layers. The validity of this purely irrotational theory can be judged by comparison with the exact solutions of Navier-Stokes equations. Here we show that our purely irrotational theory is in remarkably good agreement with the exact solution in linear analysis of the capillary instability of a viscoelastic liquid cylinder.
Keywords: Capillary instability, Viscoelastic potential flow, Additional pressure contribution, Dissipation method
(18)
J. Wang, D. D. Joseph and T. Funada. 2005.
Pressure corrections for potential flow analysis of capillary instability of
viscous fluids,
J. Fluid
Mech., 522, 383-394.
Abstract
Funada & Joseph (2002) analyzed capillary instability assuming that the flow is irrotational but the fluids are viscous (viscous potential flow, VPF). They compared their results with the exact normal mode solution of the linearized Navier-Stokes equations (fully viscous flow, FVF) and with the irrotational flow of inviscid fluids (inviscid potential flow, IPF). They showed that the growth rates computed by VPF are close to the exact solution when Reynolds number is larger than O(10) and are always more accurate than those computed using IPF. Recently, Joseph & Wang (2004) presented a method for computing a viscous correction of the irrotational pressure induced by the discrepancy between non-zero irrotational shear stress and the zero shear stress boundary condition at a free surface. The irrotational flow with a corrected pressure is called viscous correction of VPF (VCVPF). Here we compute the pressure correction for capillary instability in cases in which one fluid is viscous and the other fluid is a gas of negligible density and viscosity. The growth rates computed using VCVPF are in remarkably good agreement with the exact solution FVF.
(19)
T. S. Lundgren and D. D. Joseph. 2005.
Capillary Collapse and Rupture.
Abstract
The breakup of a liquid capillary filament is analyzed as a viscous potential flow near a stagnation point on the centerline of the filament towards which the surface collapses under the action of surface tension forces. We find that the neck is of parabolic shape and its radius collapses to zero in a finite time; the curvature at the throat tends to zero much faster than the radius, leading ultimately to a microthread of nearly uniform radius. During the collapse the tensile stress due to viscosity increases in value until at a certain finite radius, which is about 1.5 microns for water in air, the stress in the throat passes into tension, presumably inducing cavitation there.
(20)
J. C. Padrino, D. D. Joseph, T. Funada, J. Wang and W. A. Sirignano. 2007.
Stress-induced cavitation for the streaming motion of a viscous liquid past a sphere.
J. Fluid Mech. , 578, 381-411.
Abstract
The theory of stress-induced cavitation is applied here to the problem of cavitation of a viscous liquid in the streaming flow past a stationary sphere. The theory is a revision of the pressure theory which states that a flowing liquid will cavitate when and where the pressure drops below a cavitation threshold, or breaking strength, of the liquid. In the theory of stress-induced cavitation the liquid will cavitate when and where the maximum tensile stress exceeds the breaking strength of the liquid. For example, liquids at atmospheric pressure which cannot withstand tension will cavitate when and where additive tensile stresses due to motion exceed one atmosphere. A cavity will open in the direction of the maximum tensile stress which is 45o from the plane of shearing in pure shear of a Newtonian fluid. This maximum tension criterion is applied here to analyze the onset of cavitation for the irrotational motion of a viscous fluid, the special case imposed by the limit of very low Reynolds numbers and the fluid flow obtained from the numerical solution of the Navier-Stokes equations. The analysis leads to a dimensionless expression for the maximum tensile stress as a function of position which depends on the cavitation and Reynolds numbers. The main conclusion is that at a fixed cavitation number the extent of the region of flow at risk to cavitation increases as the Reynolds number decreases. This prediction that more viscous liquids at a fixed cavitation number are at greater risk to cavitation seems not to be addressed, affirmed or denied, in the cavitation literature known to us.
(21)
J. Wang and D. D. Joseph. 2006.
Purely irrotational theories of the effect of the viscosity on the decay of free gravity waves,
J. Fluid Mech., 559, 461-472.
. SHORT version with less graphs
. LONG version with more graphs
Abstract
A purely irrotational theory of the effect of viscosity on the decay of free gravity waves is derived and shown to be in excellent agreement with Lamb's (1932) exact solution. The agreement is achieved for all waves numbers k excluding a small interval around a critical k=kc where progressive waves change to monotonic decay. Very detailed comparisons are made between the purely irrotational and exact theory.
(22)
J. Wang and D. D. Joseph. 2005.
The lift, drag and torque on an airfoil in foam modeled by the potential flow of a second-order fluid.
Abstract
We compute the irrotational streaming flow of a second-order fluid past a Joukowski airfoil. The pressure and extra stress are evaluated using the irrotational flow theory, and the lift, drag and torque on the airfoil are obtained by integration of the normal stress over the surface of the airfoil. Our calculation can give rise to a lift force opposite to what would be predicted from the classical theory of aerodynamics. The result is in qualitative agreement with the experiments of the flow of a foam past an airfoil by Dollet, Aubouy and Graner 2004.
(23)
T. Funada, M. Saitoh, J. Wang and D. D. Joseph. 2005.
Stability of a Liquid Jet into Incompressible Gases and Liquids: Part 2. Effects of the
irrotational viscous pressure,
Int. J. Multiphase Flow, 31, 1134-1154.
Abstract
In this paper we investigate the effects of an irrotational, viscous pressure on the stability of a liquid jet into gases and liquids. The analysis extends the work of Funada, Joseph & Yamashita 2004 [3] in which the stability of the viscous jet was studied assuming that the motion and pressure are irrotational and the viscosity enters through the jump in the viscous normal stress in the normal stress balance at the interface. The liquid jet is always unstable; at high Weber numbers the instability is dominated by capillary instability; at low W the instability is dominated by Kelvin-Helmholtz (KH) waves generated by pressures driven by the discontinuous velocity. In the irrotational analysis the viscosity is important but the effects of shear are neglected. In fact a discontinuous velocity is not compatible with the continuity of the tangential components of velocity and shear stress so that KH instability is not properly posed for exact study using the no-slip condition but some of the effects of viscosity can be ascertained using viscous potential flow. The theory developed by Funada et al. 2004 is called viscous potential flow (VPF). Here we develop another irrotational theory in which the discontinuities in the irrotational tangential velocity and shear stress are eliminated in the global energy balance by selecting viscous contributions to the irrotational pressure. These pressures generate a hierarchy of potential flows in powers of the viscosity, but only the first one, linear in viscosity, in the irrotational viscous stress, is thought to have physical significance. The tangential velocity and shear stress in an irrotational study cannot be made continuous, but the effects of the discontinuous velocity and stress in the mechanical energy balance can be removed 'in the mean'.This theory with the additional viscous pressure is called VCVPF, viscous correction of VPF. VCVPF is VPF with the additional pressures. The theory here cannot be compared with an exact solution, which would not allow the discontinuous velocity and stress. In other problems, like capillary instability, in which VCVPF can be compared with an exact solution (Wang, Joseph & Funada JFM 2005,[4] Phys Fluid 2005,[5] JNNFM 2005[6]) the agreements are uniformly excellent in the wave number when one of the fluids is gas and in good but not uniform, agreement when both fluids are liquids.
Keywords: VCVPF (Viscous Correction of Viscous Potential Flow), Kelvin-Helmholtz (KH) Instability, Capillary Instability
(24)
T. Funada, J. Wang, D. D. Joseph, N. Tashiro and Y. Sonoda. 2005.
Solution of Mathieu’s equation by Runge-Kutta integration.
Abstract
In this note we shall show that Mathieu's equation for x(t)
may be efficiently and accurately integrated by the Runge-Kutta (RK) method under the initial conditions
for the even Mathieu functions cen(t, q) → cos (nt) as q → 0
and
for the odd Mathieu functions sen(t, q) → sin (nt) as q → 0.
(25)
J. Wang and D. D. Joseph. 2005.
Irrotational analysis of the toroidal bubble in a viscous fluid.
Abstract
We consider the problem of the rise of a toroidal gas bubble previously considered by Pedley (1968). We add the irrotational viscous drag to the force wrench in the impulse equation. In this case, the impulse equation and the energy equation governing the rise of the bubble are the same. The solution of this equation is computed; after a transient state the system evolves to a steady state in which the diameter, toroidal radius and rise velocity are constant.
(26)
J. Wang, D. D. Joseph and T. Funada. 2005.
Viscous contributions to the pressure for potential flow analysis of capillary instability of two viscous fluids,
Phys. Fluids, 17, 052105.
Abstract
Capillary instability of a liquid cylinder immersed in another liquid is analyzed based on potential flow solutions. The growth rate of the instability is obtained by considering the normal stress balance at the interface. We derive a viscous correction of the irrotational pressure which presumably arises from a boundary layer induced by the discontinuity of the tangential velocity and shear stress at the interface evaluated using the potential flow solution. We include the viscous irrotational stress and pressure correction in the normal stress balance and compare the computed growth rates to the growth rates of the exact viscous flow solution. The agreement is excellent when one of the liquids is a gas; for two viscous liquids, the agreement is good to reasonable for the maximum growth rates but poor for long waves. Calculations show that good agreement is obtained when the vorticity is relatively small or the irrotational part is dominant in the exact viscous solution. We show that the irrotational viscous flow with pressure corrections gives rise to exactly the same dispersion relation as the dissipation method in which no pressure at all is required and the viscous effect is accounted for by evaluating the viscous dissipation using the irrotational flow.
(27)
F. Viana, T. Funada, D. D. Joseph, N. Tashiro and Y. Sonoda. 2005.
Potential flow of a second-order fluid over a tri-axial ellipsoid,
J. App. Math., Issue 4, pp. 341-364.
Abstract
The problem of potential flow of a second-order fluid around an ellipsoid is solved, following general expressions in Lamb (1993), and the flow and stress fields are computed. The flow fields are determined by the harmonic potential but the stress fields depend on viscosity and the parameters of the second-order fluid. The stress fields on the surface of a tri-axial ellipsoid depend strongly on the ratios of principal axes and are such as to suggest the formation of gas bubble with a round flat nose and two-dimensional cusped trailing edge. A thin flat trailing edge gives rise to a large stress which makes the thin trailing edge thinner.
Keywords: Potential flow, Second-order fluid, Cusp, Normal extensional stress
(28)
D. D. Joseph and J. Wang. 2005.
The motion of a spherical gas bubble in viscous potential flow.
Abstract
A spherical gas bubble accelerates to steady motion in an irrotational flow of a viscous liquid induced by a balance
of the acceleration of the added mass of the liquid with the Levich drag. The equation of rectilinear motion is linear
and may be integrated giving rise to exponential decay with a decay constant 
where 
is the kinematic viscosity of the liquid and a is the
bubble radius. The problem of decay to rest of a bubble moving initially when
the forces maintaining motion are inactivated and the acceleration of a bubble
initially at rest to terminal velocity are considered. The equation of motion follows
from the assumption that the motion of the viscous liquid is irrotational. It is an elementary example of how potential
flows can be used to study the unsteady motions of a viscous liquid suitable
for the instruction of undergraduate students. Another example, considered
here, is the purely radial irrotational motion of a
viscous liquid associated with the motions of a spherical gas bubble. This gives
rise to an exact potential flow solution of the Navier-Stokes
equations in which the jump of the viscous component of the normal stress is
evaluated on the potential flow. The equation of motion for the liquid is
almost always called the Reyleigh-Plesset equation
but the viscous terms were introduced by Poritsky
(1951) and not by Plesset (1949). We show that when
the normal stress equation is converted into energy equation in the
conventional way used for inviscid fluid, the viscous
normal stress term is converted into the viscous dissipation in the liquid
evaluated on potential flow.
(29)
T. Funada, J. Wang, D. D. Joseph and N. Tashiro. 2005.
Irrotational Faraday Waves on a Viscous Fluid.
Abstract
An analysis
of irrotational Faraday waves on an inviscid fluid was given by Benjamin and Ursell 1954. Here we extend the analysis of the same
problem to purely irrotational waves on a viscous fluid. Following our earlier
work on free surface problems, two irrotational theories are presented. In the
first theory (VPF) the effects of viscosity enter only through the viscous
normal stress term evaluated on the potential. In the second irrotational
theory (VCVPF), a viscous contribution is added to the Bernoulli pressure;
otherwise the second theory is the same as the first. The second theory VCVPF
gives rise to the same damped Mathieu equation as the dissipation method.
Pressure fields are not required and not used in the dissipation method. The dissipation
method is a purely irrotational theory, though it depends on viscosity, in
which only irrotational velocity fields 
are needed. The two
purely irrotational theories VPF and VCVPF are not restricted to small
viscosities; they are restricted to small vorticity
and do not apply near no-slip wall where Vorticity is generated.
Our VCVPF
and dissipation theories give the same damped Mathieu equation as the
phenomenological approximation of Kumar and Tuckerman 1994. The damping term in
VCVPF is twice the damping rate of VPF. The growth rates of unstable
disturbances computed by VPF are uniformly larger than those computed by VCVPF
(or equivalently by Kumar and Tuckerman). Comparisons with the exact solution
and the Rayleigh-Taylor instability show that thresholds and growth rates for
viscously damped waves are better described by VPF than VCVPF.
••2006••
(30)
T. Funada, J. Wang and D. D. Joseph. 2006.
Viscous potential flow analysis of stress-induced cavitation in an aperture flow,
Atomization and Sprays, Issue 7, vol.16, pp.xx-yy.
Abstract
Cavitation in an aperture flow in a flat plate is studied using viscous potential flow. The maximum tension criterion for cavitation used here was proposed by Joseph 1995, 1998: "Liquids at atmospheric pressure which cannot withstand tension will cavitate when and where tensile stresses due to motion exceed one atmosphere. A cavity will open in the direction of the maximum tensile stress which is 45o from the plane of shearing in pure shear of a Newtonian fluid." The aperture flow is expressed using a complex potential and the stress is calculated using viscous potential flow. We find that the viscous stress is huge near the tips of the aperture, thus cavitation could be induced.
(31)
T. Funada, D. D. Joseph, M. Saitoh and S. Yamashita. 2006.
Liquid jet in a high Mach number air stream,
Int. J. Multiphase Flow, 32, 20-50.
Abstract
The instability of circular liquid jet immersed in a coflowing high velocity airstream is studied assuming that the flow of the viscous gas and liquid is irrotational. The basic velocity profiles are uniform and different. The instabilities are driven by Kelvin - Helmholtz instability due to a velocity difference and neckdown due to capillary instability. Capillary instabilities dominate for large Weber numbers. Kelvin -Helmholtz instability dominates for small Weber numbers. The wave length for the most unstable wave decreases strongly with the Mach number and attains a very small minimum when the Mach number is somewhat larger than one. The peak growth rates are attained for axisymmetric disturbances (n = 0) when the viscosity of the liquid is not too large. The peak growth rates for the first asymmetric mode (n = 1) and the associated wave length are very close to the n = 0 mode; the peak growth rate for n = 1 modes exceeds n = 0 when the viscosity of the liquid jet is large. The effects of viscosity on the irrotational instabilities are very strong. The analysis predicts that breakup fragments of liquids in high speed air streams may be exceedingly small, especially in the transonic range of Mach numbers.
Keywords: Capillary instability; Kelvin - Helmholtz instability; Isentropic compressible gas; Viscous potential flow; Irrotational flow of viscous fluids
(32)
D. D. Joseph. 2006.
Potential Flow of Viscous Fluids: Historical Notes,
Int. J. Multiphase Flow, 32, 285-310.
Abstract
In this note I will attempt to identify the main events in the history of thought about irrotational flow of viscous fluids. I am of the opinion that when considering irrotational solutions of the Navier-Stokes equations it is never necessary and typically not useful to put the viscosity to zero. This observation runs counter to the idea frequently expressed that potential flow is a topic which is useful only for inviscid fluids; many people think that the notion of a viscous potential flow is an oxymoron. Incorrect statements like "... irrotational flow implies inviscid flow but not the other way around" can be found in popular textbooks.
Though convenient, phrases like "inviscid potential flow" or "viscous potential flow" confuse properties of the flow (potential or irrotational) with properties of the material (inviscid, viscous or viscoelastic); it is better and more accurate to speak of the irrotational flow of an inviscid or viscous fluid.
(33)
J. Wang and D. D. Joseph. 2006.
Pressure corrections for the effects of viscosity on the irrotational flow outside Prandtl's boundary layer,
J. Fluid Mech., 557, 145-165.
Abstract
In this work, we try to understand the viscous effects of the outer potential flow on Prandtl's boundary layer. For a body moving with a constant velocity in an otherwise quiescent liquid, the non-zero viscous dissipation of the outer potential flow gives rise to an additional drag, increasing the drag calculated from the boundary layer flow alone. The discrepancy of the shear stress at the outer edge of the boundary layer induces a viscous pressure correction of the irrotational pressure. The pressure correction varies inside the boundary layer and has viscous effects on the body, which are not captured in Prandtl's boundary layer theory. These viscous effects of the outer flow on Prandtl's boundary layer is small when the Reynolds number is high.
(34)
J. Wang and D. D. Joseph. 2006.
Boundary layer analysis for effects of viscosity of the irrotational flow on the flow induced by a rapidly rotating cylinder in a uniform stream,
J. Fluid Mech., 557, 167-190.
Abstract
We study the streaming flow past a
rapidly rotating circular cylinder (figure 1)1. The starting point is the full continuity and
momentum equations without any approximations. We assume that the solution is a boundary layer flow near the
cylinder surface with the potential flow outside the boundary layer. The order
of magnitude of the terms in the continuity and momentum equations can be estimated inside the boundary layer. When terms
in the order of 
and higher are
dropped, where 
is the boundary layer thickness and 
is the radius of the cylinder, the equations used
by Glauert (1957)
[2] are recovered. Glauert's solution ignores the irrotational rotary component of the flow inside the
boundary layer, which is consistent with dropping terms in the
governing equations.
We propose a new solution to this
problem, in which the velocity field is decomposed into two parts. Outside the
boundary layer, the flow is irrotational and can be
decomposed into a purely rotary flow and a potential flow past a fixed
cylinder. Inside the boundary layer, the velocity is decomposed into an irrotational purely rotary flow and a boundary
layer flow. Inserting this decomposition of the velocity field inside the
boundary layer into the governing equations, we obtain a new set of equations
for the boundary layer flow, in which we do not drop the terms in the order of or higher. The pressure can no longer be
assumed to be a constant across the boundary layer, and the continuity of shear
stress at the outer edge of the boundary layer is enforced. We
solve this new set of equations using Glauert's
method, i.e. to expand the solutions as a power series of 
, where 
is the uniform
stream velocity and 
is the circulatory velocity at the
outer edge of the boundary layer. The pressure from this boundary layer
solution has two parts, an inertia part and a viscous part. The inertial part
comes from the inertia terms in the momentum equations and is
in agreement with the irrotational pressure; the viscous part
comes from the viscous stress terms in the momentum equations and may be viewed
as a viscous pressure correction, which contributes to both drag and lift. Our
boundary layer solution is in reasonable to excellent agreement with the
numerical simulation of Padrino and Joseph (2004) [13].
(35)
J. C. Padrino and D. D. Joseph. 2006.
Numerical study of the steady state uniform flow past a rotating cylinder,
J. Fluid Mech., 557, 191-223.
Abstract
Relevant
results from the numerical solution of the two-dimensional incompressible
unsteady Navier-Stokes equations for streaming flow
past a rotating circular cylinder are presented in this study. The numerical solution of the equations of
motion is conducted with a commercial computational fluid dynamics package
which discretizes the equations applying the control
volume method. The numerical setup is
validated by comparing results for a Reynolds number based on the free stream
of Re = 200 and peripheral speed of 
= 3, 4 and 5 with
results from the literature. After the
validation stage, various pairs of Re
and are specified in order
to carry on the numerical experiments. These values are Re = 200 with
= 4 and 5; Re = 400 with = 4, 5 and 6, and Re = 1000 with = 3. In all these cases, gentle convergence to
fully developed steady state is reached. From the numerical vorticity distribution, the
position of the outer edge of the vortical region is
determined as a function of the angular coordinate. This position is found by means of a
reasonable criterion set to define the outmost curve around the cylinder where
the vorticity magnitude reaches a certain cut off
value. By considering the average value
of this profile, a uniform vortical region thickness
is specified for every pair of Re and
.
Next, the
theoretical approach of Wang and Joseph (2004a) and the numerical results are
utilized to determine two different values of the effective vortical
region thickness for every pair of Re
and . One effective
thickness 
is obtained from the
match between the additional drag on the outer edge of the vortical
region according to the viscous correction of viscous potential flow (VCVPF)
and the corresponding numerical profile while the other thickness 
is determined from the
match between the pressure lift on the cylinder obtained from Wang and Joseph
(2004a)'s simple modification of the boundary layer analysis due to Glauert (1957) and the numerical value of the pressure lift
coefficient. Each of these two values of
the effective vortical region thickness is used to
compute various parameters relevant to this type of fluid motion, namely, the
torque on the rotating cylinder; the circulatory velocity at the edge of the vortical region, which links the cylinder's angular
velocity with the circulation of the irrotational
flow of the viscous fluid outside this region, and the viscous
dissipation. For some of these
parameters, predictions from the theoretical approaches of Glauert
(1957) and Wang and Joseph (2004a) are presented for comparison. The values of both effective thicknesses, and , are found to be fairly close. Then, we show that, with the choice of the
thickness as a unique effective
thickness, the simple modification of Glauert's
boundary layer analysis and the VCVPF applied to balance the shear stress
discrepancy at the outer edge of the vortical region
as proposed by Wang and Joseph (2004a) lead to expressions that exhibit better
general agreement with the numerical results than Glauert's
solution.
(36)
J. C. Padrino, T. Funada and D. D. Joseph. 2006.
Purely irrotational theories for the viscous effects on the oscillations of drops and bubbles.
. SHORT version
Abstract
In this paper, we apply two purely irrotational theories of the motion of a viscous fluid, namely, viscous potential flow (VPF) and the dissipation method to the problem of the decay of waves on the surface of a sphere. We treat the problem of the decay of small disturbances on a viscous drop surrounded by gas of negligible density and viscosity and a bubble immersed in a viscous liquid. The instantaneous velocity field in the viscous liquid is assumed to be irrotational. In VPF, viscosity enters the problem through the viscous normal stress at the free surface. In the dissipation method, viscosity appears in the dissipation integral included in the mechanical energy equation. Comparisons of the eigenvalues from VPF and the dissipation approximation with those from the exact solution of the linearized governing
equations are presented. The results show that the viscous irrotational theories exhibit most of the features of the wave dynamics described by the exact solution. In particular, VPF and DM give rise to a viscous correction for the frequency that determines the crossover from progressive to standing waves. Good to reasonable quantitative agreement with the exact solution is also shown for certain ranges of modes and dimensionless viscosity: For large viscosity and short waves, VPF is a very good approximation to the exact solution. For small viscosity and long waves, the dissipation method furnishes the best approximation.
. LONG version
Abstract
Two purely irrotational theories of the motion of viscous fluids, viscous potential flow (VPF) and a viscous correction of viscous potential flow (VCVPF), have recently been applied to study capillary instability and to obtain the rate of decay of free gravity waves. The results from these analyses are, in general, in good to excellent agreement with exact solutions. Furthermore, the application of the dissipation method to these problems gives the same results as VCVPF. In this paper, we apply the VPF and VCVPF approximations as well as the dissipation method to the problem of the decay of waves on spheres. We treat the problem of the decay of small disturbances on a viscous drop surrounded by gas of negligible density and viscosity and a bubble immersed in a viscous liquid. The instantaneous velocity field in the viscous liquid is assumed to be irrotational. The linearized governing equations are solved by normal modes. The dissipation method applied to this problem gives rise to the same dispersion relation as VCVPF. Detailed comparisons of the eigenvalues from VPF and VCVPF and the exact solution are presented. Good agreement with the exact solution for the two purely irrotational solutions is demonstrated.
Key words: drops, bubbles, potential flow, two-phase flow, gas-liquid flow, viscous potential flow.
(37)
T. Funada. 2006.
Funada's file of potential functions for ellipsoids.
(38)
D. D. Joseph and J. C. Padrino. 2006.
Irrotational viscous pressure and the dissipation method.
(39)
J. Wang, T. Funada and D. D. Joseph. 2006.
Irrotational motions of bubbles under the action of acceleration of added mass and viscous drag.
. Abstract
We present elementary examples of how irrotational flows can be used to study the unsteady motions of bubbles in a viscous liquid suitable for the instruction of undergraduate students. A spherical gas bubble accelerates to steady motion in an irrotational flow of a viscous liquid induced by a balance of the acceleration of the added mass of the liquid with the viscous drag. The equation of rectilinear motion is linear and may be integrated giving rise to exponential decay with a decay constant 18 v t/a2 where v is the kinematic viscosity of the liquid and a is the bubble radius. The problem of decay to rest of a bubble moving initially when the forces maintaining motion are inactivated and the acceleration of a bubble initially at rest to terminal velocity are considered. We also discuss the motion of a massless cylindrical bubble under the combined action of Kutta-Joukowski lift, acceleration of added mass and viscous drag. The cylinder moves with a constant speed along a circular path if the liquid is inviscid, whereas it goes to rest in a spiral fashion when the viscous drag is added.
(40)
J. C. Padrino and D. D. Joseph. 2007.
Correction of Lamb's dissipation calculation for the effects of viscosity on capillary-gravity waves.
Phys. Fluids,19, 082105.
. Abstract
Purely irrotational theories of the flow of a viscous liquid are applied to model the effect of viscosity on the decay and oscillation of capillary-gravity waves. In particular, the dissipation approximation used in this analysis gives rise to a viscous correction of the frequency of the oscillations which was not obtained by Lamb's (1932) dissipation calculation. Moreover, our dissipation method goes beyond Lamb's in the sense that it yields an eigenvalue relation valid for the entire continuous spectrum of wave numbers. Comparisons are presented between the purely irrotational theories and Lamb's exact solution, showing good to reasonable agreement for long, progressive waves and for short, standing waves, even for very viscous liquids. The performance of the irrotational approximations deteriorates within an interval of wave-numbers containing the cut-off where traveling waves become standing ones.
(41)
D. D. Joseph, T. Funada and J. Wang. 2006.
Potential Flows of Viscous and Viscoelastic Fluids. (Book)
CAMBRIDGE UNIVERSITY PRESS, IN PRODUCTION.
Part (2).
Part (3).
Part (4).
(42)
D. D. Joseph. 2006.
Helmholtz decomposition coupling rotational to irrotational flow of a viscous fluid.
14272-14277 | PNAS | September 26, 2006 | Vol. 103 | No.39. http://www.pnas.org/cgi/doi/10.1073/pnas.0605792103.
. Abstract
In this work, I present the form of the Navier-Stokes equations implied by the Helmholtz decomposition in which the relation of the irrotational and rotational velocity fields is made explicit. The idea of self-equilibration of irrotational viscous stresses is introduced. The decomposition is constructed by first selecting the irrotational flow compatible with the flow boundaries and other prescribed conditions. The rotational component of velocity is then the difference between the solution of the Navier-Stokes equations and the selected irrotational flow. To satisfy the boundary conditions, the irrotational field is required, and it depends on the viscosity. Five unknown fields are determined by the decomposed form of the Navier-Stokes equations for an incompressible fluid: the rotational component of velocity, the pressure, and the harmonic potential. These five fields may be readily identified in analytic solutions available in the literature. It is clear from these exact solutions that potential flow of a viscous fluid is required to satisfy prescribed conditions, like the no-slip condition at the boundary of a solid or continuity conditions across a two-fluid boundary. It can be said that equations governing the Helmholtz decomposition describe the modification of irrotational flow due to vorticity, but the analysis shows the two fields are coupled and cannot be completely determined independently.
(43)
S. Dabiri, W. A. Sirignano, and D. D. Joseph. 2007.
Cavitation in an Orifice Flow. Phys. Fluids. 19, 072112.
. Abstract
The purpose of this study is to identify the potential locations for cavitation induced by total stress on the flow of a liquid through and orifice of an atomizer. A numerical simulation of two-phase incompressible flow is conducted in an axisymmetric geometry of the orifice for Reynolds number between 100 and 2000. The orifice has a rounded upstream corner and a sharp downstream corner with length-to-diameter ratio between 0.1 and 5. The total stress including viscous stress and pressure has been calculated in the flow field and , from there, the maximum principal stress is found. The total-stress criterion for cavitation is applied to find the regions where cavitation is likely to occur and compared with those of the traditional pressure criterion. Results show that the viscous stress has significant effects on cavitation. The effect of geometry and occurrence of hydraulic flip in the orifice on the total stress is studied. The Navier-Stokes equations are solved numerically using a finite-volume method and a boundary-fitted orthogonal grid that comes from the streamlines and potential lines of an axisymmetric equipotential flow in the same geometry. A level-set formulation is used to track the interface and model the surface tension.
(44)
S. Dabiri, W. A. Sirignano, and D. D. Joseph. 2008.
Two-Dimensional and Axisymmetric Viscous Flow in Apertures.
J. Fluid Mech. In Press.
. Abstract
The flow in a plane liquid jet from an aperture is obtained by direct simulation of the Navier-Stokes equations. The gas-liquid interface is tracked using level set method. Flows are calculated for different Reynolds and Weber numbers. When We = &infin the maximum value of the discharge coefficient appears around Re =O(100). The regions that are vulnerable to cavitation due to the total stress are identified from calculations based on Navier-Stokes equations and viscous potential flow; the two calculations yield similar results. We prove that the classical potential flow solution does not give rise to a normal component of the rate of strain at the free streamline. Therefore, that the normal component of the irrotational viscous stresses also vanishes and cannot change the shape of the free surface. The results of calculations of flows governed by the Navier-Stokes equations are close to those for viscous potential flow outside the vorticity layers at solid boundaries. The Navier-Stokes solutions for the axisymmetric aperture are also given for two values of Reynolds numbers. The results for axisymmetric and planar apertures are qualitatively similar, but the axisymmetric apertures have a lower discharge coefficient and less contraction.
(45)
D. D. Joseph and T. Funada. 2006.
Potential Flow of A Cylindrical Vortex Sheet in A Viscous Fluid.
(46)
J. C. Padrino, 2007.
Irrotational disturbances of the motion of a viscous fluid. Short Communication. Dept. Aerospace Engineering and Mechanics. University of Minnesota, Twin Cities. Posted 24 March 2008
(47)
J. C. Padrino, 2006.
Shear instability of a planar liquid jet immersed in a high speed gas stream - Viscous Potential Flow analysis. Internal Report. Dept. Aerospace Engineering and Mechanics. University of Minnesota, Twin Cities. Posted 24 March 2008
(48)
J. C. Padrino and D. D. Joseph. 2008.
Viscous irrotational theories and the force on an expanding bubble-A cell model analysis..
Under consideration of Industrial & Engineering Chemistry Research
. Abstract
The dynamics of a bounded viscous incompressible fluid surrounding a spherical bubble in rectilinear motion simultaneously experiencing volume changes is examined by means of two viscous irrotational theories, namely, viscous potential flow and the dissipation method. The forces that the liquid produces on the bubble and on the outer spherical boundary of the liquid are determined from these two approaches at the instant when the bubble is concentric with the outer surface. Viscous potential flow involves surface integration of the irrotational normal stress; the dissipation method stems from the mechanical energy balance, including the dissipation integral, evaluated in potential flow. In the inner boundary, zero tangential stress is enforced. Two choices for the tangential stress condition on the outer boundary are considered: Zero tangential stress or irrotational tangential stress. In a sense, this is an extension to include viscous effects of the inviscid analysis by Sherwood [Int. J. Multiphase Flow, 25, 705, 1999]. The potential flow that follows from Sherwood's work is used in the derived formulae to compute the drag. To the added-mass forces associated with the bubble acceleration and rate of change of the bubble radius determined by Sherwood, a viscous contribution is added here that depends upon the instantaneous bubble velocity and the inner and outer instantaneous radii of the bubble-liquid cell. When the outer radius is taken to infinity, the expressions for the drag yield results given in the literature. If the inner and outer radii are held fixed, results from the cell model may be used to approximate the drag on a bubble moving in a bubbly flow with the same volume fraction as the cell. The analysis yields two results for the viscous drag on the bubble contingent on the boundary condition applied on the outer sphere. These formulas have been presented in the literature, although regarded as contradictories. By emphasizing the role of the tangential stress on the outer boundary, it is shown that both results are valid as they depend on the choice of the outer dynamic boundary condition. These results agree to first order in the volume fraction. The terminal rise velocity of a bubble swarm is derived using the drag from the viscous irrotational theories. Results for the drag coefficient and bubble rise velocity are compared with other theoretical results as well as data from numerical simulations and experiments.
(49)
A. M. Ardekani, R. H. Rangel and D. D. Joseph. 2007.
Motion of a sphere normal to a wall in a second-order fluid .
J. Fluid Mech. 587,163-172.
. Abstract
The motion of a sphere normal to a wall is investigated. The normal stress at the surface of the sphere is calculated and the viscoelastic effects on the normal stress for different separation distances are analysed. For small separation distances, when the particle is moving away from the wall, a tensile normal stress exists at the trailing edge if the fluid is Newtonian, while for a second-order fluid a larger tensile stress is observed. When the particle is moving towards the wall, the stress is compressive at the leading edge for a Newtonian fluid whereas a large tensile stress is observed for a second-order fluid. The contribution of the second-order fluid to the overall force applied to the particle is towards the wall in both situations. Results are obtained using Stokes equations when \alpha_1 + \alpha_2 = 0. In addition, a perturbation method has been utilized for a sphere very close to a wall and the effect of a non-zero \alpha_1 + \alpha_2 is discussed. Finally, viscoelastic potential flow is used and the results are compared with the other methods.
(50)
A. M. Ardekani, R. H. Rangel and D. D. Joseph. 2008.
Two spheres in a free stream of a second-order fluid. Under consideration of
Phys. Fluids.
. Abstract
The forces acting on two fixed spheres in a second-order uniform flow are investigated. When \alpha_1 +alpha_2 = 0, where \alpha_1 and \alpha_2 are fluid parameters related to the first and second normal stress coefficients, the velocity field for a second-order fluid is the same as the one predicted by the Stokes equations while the pressure is modified. The Stokes solutions given by Stimson and Jeffery [Proc. R. Soc. London, Ser. A 111, 110 (1926)] for the case when the flow direction is along the line of centers and Goldman et al.[Chemical Eng. Science 21, 1151 (1966)] for the case when the flow direction is perpendicular to the line of centers are utilized and the stresses and the forces acting on the particles in a second-order fluid are calculated. For flow along the line of centers or perpendicular to it, the net force is in the direction that tends to decrease the particle separation distance. For the case of flow at arbitrary angle, unequal forces are applied to the spheres perpendicularly to the line of centers. These forces result in a change of orientation of the sedimenting spheres until the line of centers aligns with the flow direction. In addition, the potential flow of a second-order fluid past two fixed spheres in uniform flow is investigated. The normal stress at the surface of each sphere is calculated and the viscoelastic effects on the normal stress for different separation distances are analyzed. The contribution of the potential flow of a second-order fluid to the force applied to the particles is an attractive force. Our explanations of the aggregation of particles in viscoelastic fluids rest on three pillars; the first is a viscoelastic "pressure" generated by normal stresses due to shear. Secondly, the total time derivative of the pressure is an important factor in the forces applied to moving particles. The third is associated with a change in the normal stress at points of stagnation which is a purely extensional effect unrelated to shearing.
(51)
S. Dabiri, A. M. Ardekani, W. A. Sirignano and D. D. Joseph. 2008.
Disintegration of moving liquid sheets using viscous potential flow.
In preparation.
. Abstract
The breakup of a thin liquid film moving in a second fluid utilizing viscous potential analysis and considering disjoining pressure is studied. The occurrence of cavitation due to local pressure drop in the film has been considered. For a large Weber number and density ratio, cavitation might occur and it could be the main reason for breakup.
(52)
H. Kim, S. Kwon, J.C. Padrino and T. Funada, 2007.
Viscous potential flow analysis of capillary instability with heat and mass transfer.
In preparation.
. Abstract
We carry out the linear viscous-irrotational analysis of capillary instability with heat transfer and phase change. We consider the cylindrical interface shared by two viscous incompressible fluids enclosed by two concentric cylinders. In viscous potential flow, viscosity enters the model through the balance of normal stresses at the interface. We write the dispersion relation from the stability analysis for axisymmetric disturbances in terms of a set of dimensionless numbers that arise in this phase change problem. For the film boiling condition, plots depicting the effect of some of these parameters on the maximum growth rate for unstable perturbations and critical wavenumber for marginal stability are presented and interpreted. Viscous effects of a purely irrotational motion in the presence of heat and mass transfer can stabilize an otherwise unstable gas-liquid interface.